6 Tips for Mental Math Mastery for Elementary Students and Beyond

For many children, mathematics can be a challenging subject to grasp. The difficulty lies in the abstract nature of math concepts, which often require strong cognitive skills, patience, and an ability to visualize problems.

Children may struggle with basic math due to a variety of reasons, may have trouble with basic math for different reasons. They might not be ready to learn, they could have trouble understanding the words used, or they might not grasp the basic ideas yet.

Ignoring these early signs of struggle, under the assumption that children are simply "too young" to understand, is a common but significant mistake.

Possible Consequences of Overlooking Children’s Math Difficulties:

• Child Develops a Fixed Mindset: Children may start to view themselves as "bad at math," which can become a significant hurdle.
• Decreased Engagement: Believing they can't improve, they may lose interest in math and avoid it altogether.
• Cycle of Frustration: Without foundational support, children may experience ongoing frustration and self-doubt.
• Increased Complexity: As math concepts build upon earlier skills, a weak foundation makes new concepts harder to understand.
• Growing Aversion: This can lead to greater confusion and a deeper dislike of math as they progress in school.

But, math isn't everything, right? Well...

Math skills are essential not only for succeeding in school but also for functioning in everyday life.

Like the child’s ability to understand how much money to give and/or take, time management, and logical decision-making.

Not to mention the fact that math-related subjects are critical in an increasing number of careers.

What can we do about it?

Once you recognize that your child is struggling to understand basic elementary math, you must address it immediately; procrastinating on this is doing a disservice to your child.

5 Tips on Mastering Elementary Math

Here's how:

As you can see in the illustration above, this tactic requires mastering multiplication by 10, which is simple, just add a zero at the end to the other factor (other number).

Let's say you have to solve this equation: 45 multiplied by 9

Instead of counting '45, 90, 135, ...' until you get your answer, or using pen and paper to do long multiplication, it's easier and simpler to multiply 45 by 10 and then subtract 45.

Because of the rule for multiplying by 10, we know that 45 × 10 equals 450. Now, all that's left is to subtract 45 from 450, which equals 405.

To use this trick, all you need is to understand the rule for multiplying 10's.

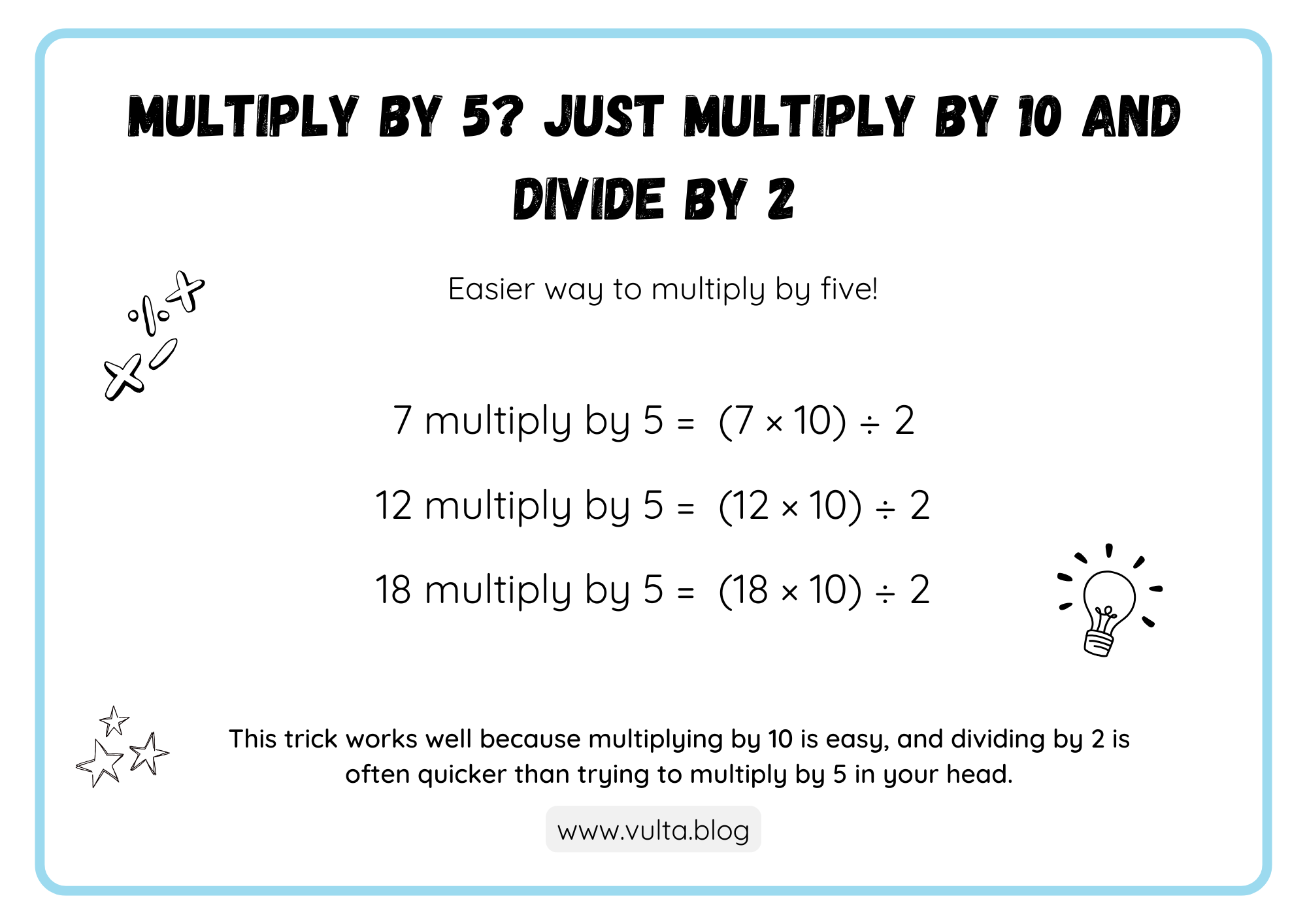
Multiplying by 5 is simple when you think of it as multiplying by 10 and then dividing by 2. This method breaks down the problem into easier steps that can be done mentally, saving time and reducing errors.

When it comes to mental math, adding and subtracting numbers like 5’s, 10’s, and 20’s is easier than calculating with certain odd numbers.

Try solving these:

Math Problem: 190 - 19 = ?

Instead of subtracting 19 directly, try subtracting 20 and then adding 1.

Math Problem: 250 - 49 = ?

Instead of subtracting 49 directly, try subtracting 50 and then adding 1.

Math Problem: 82 + 19 = ?

Instead of adding 19 directly, try adding 20 and then subtracting 1.

General Method for Multiplying Two Numbers Ending in 5 with the Same Value:

1. Start by multiplying the last digit of each number (both are 5), which always equals 25.
2. Next, add 1 to the first digit of the number (for example, 2 becomes 3, 3 becomes 4, etc.), then multiply the result by the first digit number.
3. Finally, place the result of the second step in front of the 25 from the first step to get the final answer.

This method works for any two-digit number that ends in 5 and has the same first digit. Let’s look at some examples:

To solve 25 x 25:

1. Multiply the last digit of each number (5 x 5), which always equals 25.
2. Then, add 1 to the first digit (2 + 1), and multiply the result by 2 (the first digit number): (3 x 2) = 6.
3. Finally, put the 6 in front of the 25 from step 1 to get the result: 625.

To solve 35 x 35:

1. Multiply the last digit of each number (5 x 5), which equals 25.
2. Add 1 to the first digit (3 + 1), and multiply the result by 3 (the first digit number): (4 x 3) = 12.
3. Put the 8 in front of the 25 to get the result: 1225.

To solve 65 x 65:

1. Multiply the last digit of each number (5 x 5), which equals 25.
2. Add 1 to the first digit (6 + 1), and multiply the result by 6 (the first digit number): (7 x 6) = 42.
3. Put the 14 in front of the 25 to get the result: 4225.

To solve 95 x 95:

1. Multiply the last digit of each number (5 x 5), which equals 25.
2. Add 1 to the first digit (9 + 1), and multiply the result by 9 (the first digit number): (10 x 9) = 90.
3. Put the 20 in front of the 25 to get the result: 9025.

As a general rule, if you find something difficult to calculate, try the doubling and halving tactic and see if it made it simpler to calculate.

Here are some examples:

1. 16 × 25: Halve 16 to get 8, and double 25 to get 50. Then multiply: 8 × 50 = 400.
2. 48 × 125: Halve 48 to get 24, and double 125 to get 250. Then multiply: 24 × 250 = 6000.
3. 36 × 15: Halve 36 to get 18, and double 15 to get 30. Then multiply: 18 × 30 = 540.

To simplify Complicated Problems, you have to butcher it into simpler problems.

Here's an example:

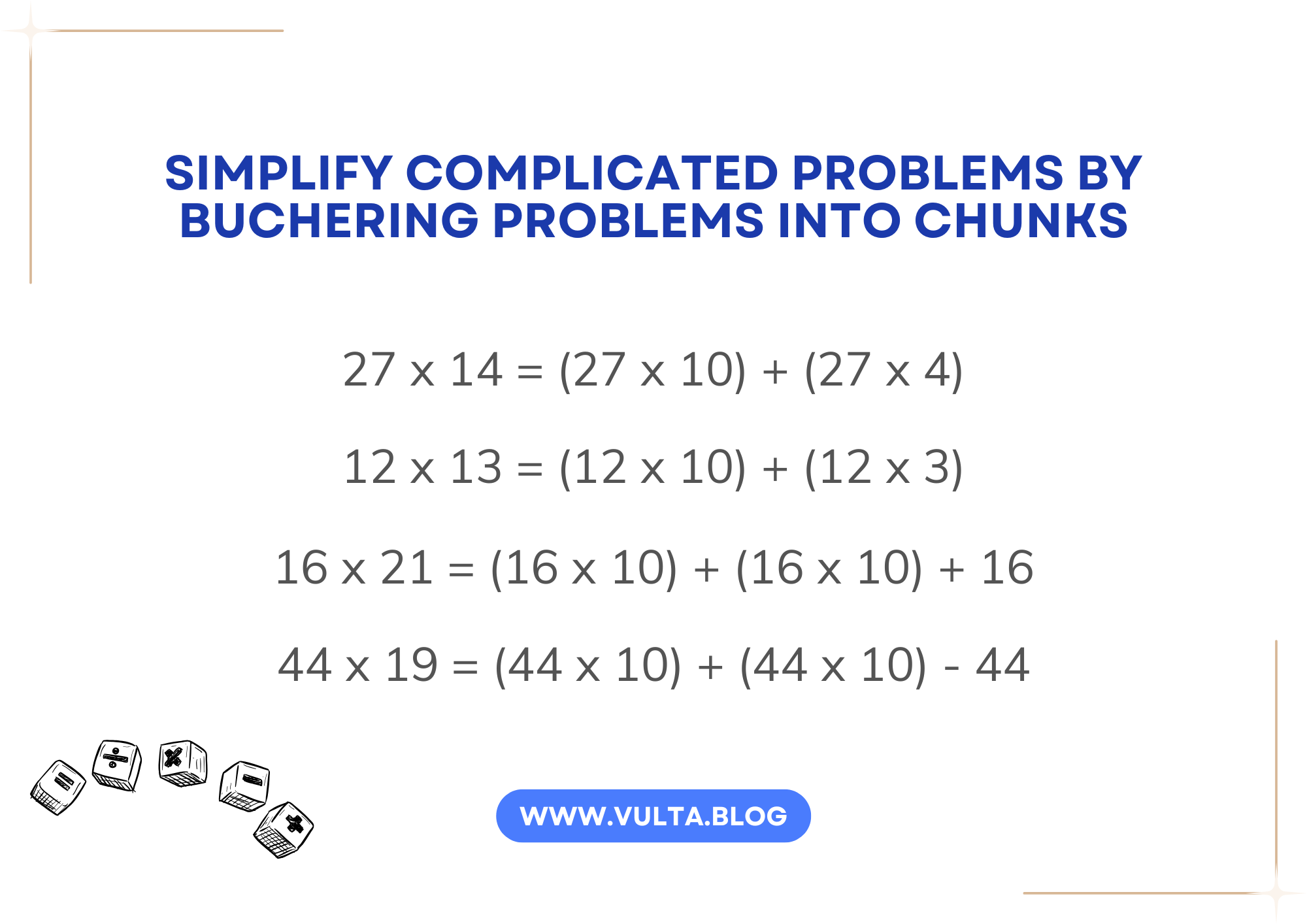
As you can see in one of the examples privded above, butchering complicated into simpler ones also gives us the opportunity to do the other mental math tactics we have discussed.